Application of Exponential Functions: Finding the Interest Rate

Example:

What is the interest rate necessary for an investment to quadruple after 7 year of continuous compound interest?

Solution:

Since this question involve continuous compound interest, we will use the associated formula.

$A=Pe^{rt}$

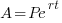
We are given that the invest quadruples in 7 years. This tells me that when t=7 that A will be 4 times P. I can write that in symbols A=4P.

Substitute these values into the continuous compound formula and solve for the interest rate.

Continuous compound formula	$A=Pe^{rt}$
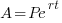Substitute the values of t and A into the formula	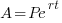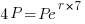$A=Pe^{rt}$ $4P=Pe^{r*7}$ $4P=Pe^{7r}$
Solve for r by dividing both sides by P and simplifying	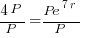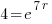$4P=Pe^{7r}$ ${4P}/P={Pe^{7r}}/P$ $4=e^{7r}$
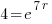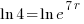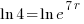Solve for r by taking the log of both sides.	$4=e^{7r}$ $ln 4=ln e^{7r}$
Solve for r by using the power rule and simplifying	$ln 4=ln e^{7r}$
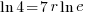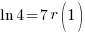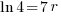Solve for r by dividing both sides by 7 and simplifying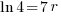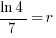
Find the value in the calculator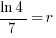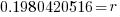
Write the answer as a percentage rounded to two decimal places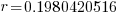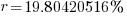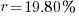

math15fun.com