2.2 Circles, 2.3 Circles, Finding Intercepts of a Circle, Intercpets

Finding the Intercepts of a Circle Touching an Axis (Tangent to an axis)

An x-intercept is where the graph touches or crosses the x-axis.

A y-intercept is where the graph touches of crosses the y-axis.

To find an x-intercept: Let y=0 and solve for x.

To find an y-intercept: Let x=0 and solve for y.

 

Example:  Find the intercepts of the circle for the given equation.

(x-1)^2+(y+2)^2=4

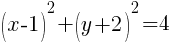
Solution:

To find an x-intercept, let y=0 and solve for x.

(x-1)^2+(y+2)^2=4

(x-1)^2+(0+2)^2=4

(x-1)^2+(2)^2=4

(x-1)^2+4=4

(x-1)^2+4-4=4-4

(x-1)^2=0

sqrt{(x-1)^2}=sqrt{0}

x-1=0

x-1+1=0+1

x=1

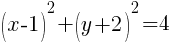
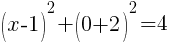
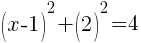
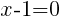
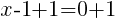
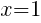
This equation has one x-intercept. 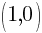(1,0)

To find a y-intercept, let x=0 and solve for y.

(x-1)^2+(y+2)^2=4

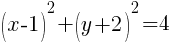
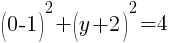
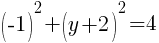
(0-1)^2+(y+2)^2=4

(-1)^2+(y+2)^2=4

1+(y+2)^2=4

(y+2)^2+1-1=4-1

(y+2)^2=3

sqrt{(y+2)^2}=sqrt{3}

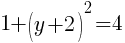
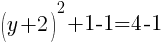
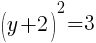
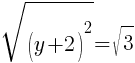
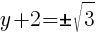
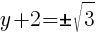
y+2=pm sqrt{3}

y+2=pm sqrt{3}

y+2-2=-2 pm sqrt{3}

y=-2 pm sqrt{3}

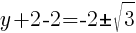
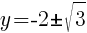
Approximately 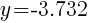y=-3.732 and x=-0.2679

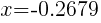
This equation has two y-intercepts. (0,-2 + sqrt{3}) and (0,-2 - sqrt{3})

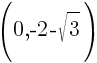
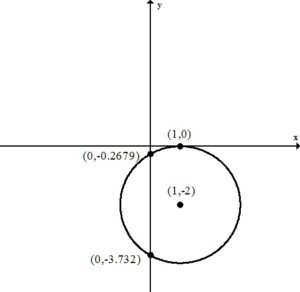
A tangent line to a circle may be defined as a line that intersects the circle in a single point.

This circle is tangent to the x-axis since it is touching the x-axis in a single point.  The x-axis (y=0) is the tangent line for the point on the circle (1,0).

Example:  Find the intercepts of the circle for the given equation.

(x-3)^2+(y-1)^2=9

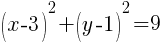
Solution:

To find an x-intercept, let y=0 and solve for x.

(x-3)^2+(y-1)^2=9

(x-3)^2+(0-1)^2=9

(x-3)^2+(-1)^2=9

(x-3)^2+1=9

(x-3)^2+1-1=9-1

(x-3)^2=8

sqrt{(x-3)^2}=sqrt{8}

x-3=sqrt{4*2}

x-3=pm 2sqrt{2}

x-3+3=3 pm 2sqrt{2}

x=3 pm 2sqrt{2}

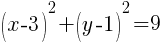
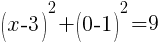
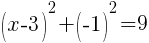
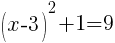
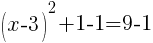
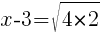
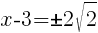
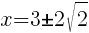
Approximately 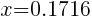x=0.1716 and x=5.828

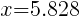
This equation has two x-intercepts. (3+2sqrt{2},0) and (3-2sqrt{2},0)

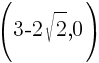
To find a y-intercept, let x=0 and solve for y.

(x-3)^2+(y-1)^2=9

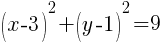
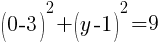
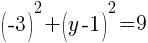
(0-3)^2+(y-1)^2=9

(-3)^2+(y-1)^2=9

9+(y-1)^2=9

(y-1)^2+9-9=9-0

(y-1)^2=0

sqrt{(y-1)^2}=sqrt{0}

y-1=0

y-1+1=0+1

y=1

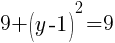
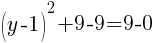
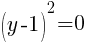
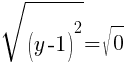
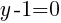
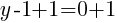
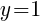
This equation has one y-intercept. 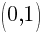(0,1).

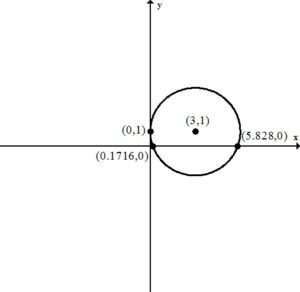
This circle is tangent to the y-axis since it is touching the y-axis in a single point.  The y-axis (x=0) is the tangent line for the point on the circle (0,1).