Example: Solve the equation.
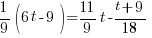
Solution:
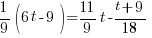 |
|
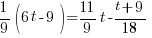 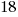 * *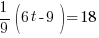 * *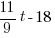 * *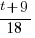 |
|
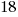 * *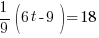 * *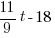 * *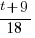 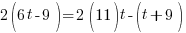 |
|
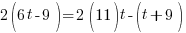 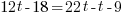 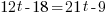 |
|
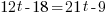 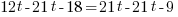 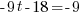 |
|
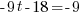 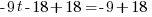 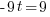 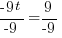 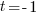 |
The solution to the equation is -1.
Here is a youtube video that might help as well.
Example: Solve the equation.
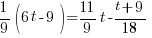
Solution:
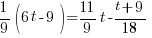 |
|
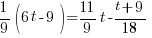  * *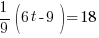 * *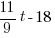 * *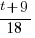 |
|
 * *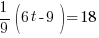 * *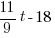 * *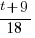 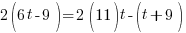 |
|
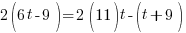 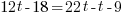 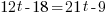 |
|
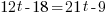 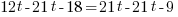 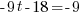 |
|
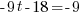 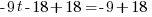 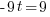 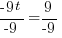 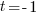 |
The solution to the equation is -1.
Here is a youtube video that might help as well.