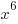3.2 Properties of a Function's Graph, 3.3 Properties of Functions

Even, Odd, or Neither

Example: Determine if the function is even, odd, or neither.

f(x)=x^7+x^6

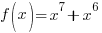
Background Knowledge:

For a function to be even it must fit the following definition.

f(- x)=f(x)

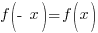
In words, this means that 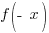f(- x) must equal the same expression as the original function.

For a function to be odd it must fit the following definition.

f(- x)=-f(x)

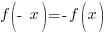
In words, this means that 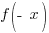f(- x) must equal the opposite expression as the original function.

We will calculate 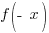f(- x)  for the given function and determine if it fits either definition.

Solution:

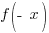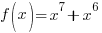We will calculate f(- x)  forf(x)=x^7+x^6 and determine if it fits either definition.

Calculate f(- x)
 f(- x)=(- x)^7+(- x)^6
Simplify (- x)^7.

  • Group the negatives. Since there are an odd number of negatives multiplied the product is negative.
  • Group the x’s.  Since the bases are the same, 7 x’s multiplied equals x^7
 f(- x)=(- x)^7+(- x)^6
f(- x)=(- x)(- x)(- x)(- x)(- x)(- x)(-x)+(- x)^6
f(- x)=-x^7+(- x)^6
Simplify (- x)^6.

  • Group the negatives. Since there are an even number of negatives multiplied the product is positive.
  • Group the x’s.  Since the bases are the same, 6 x’s multiplied equals x^6

 
f(- x)=-x^7+(- x)^6
f(- x)=-x^7+(- x)(- x)(- x)(- x)(- x)(- x)
f(- x)=-x^7+ x^6

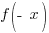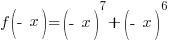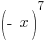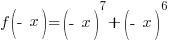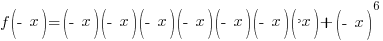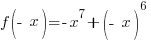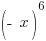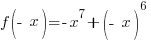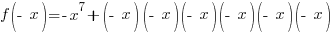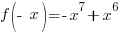Is the function even? No, 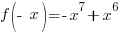 f(- x)=-x^7+ x^6 is not the same as the original function.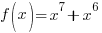 f(x)=x^7+x^6

Is the function odd? No, 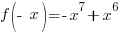f(- x)=-x^7+ x^6 is not the opposite of the original function. -f(x)=-(x^7+x^6)=-x^7-x^6

This function is not even or odd so we categorize it as “neither.”

YouTube Videos

The website of Professor Amanda Sartor

The darknet market, exemplified by Ares Market, operates on anonymity and security, offering illicit goods and services using cryptocurrency like XMR and BTC. Its focus on speed and safety attracts users seeking alternative marketplaces. See darknet markets reviews at darkfail