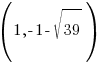Example: Classify the function as a polynomial function, rational function, or root function, and then find the domain. Write the domain interval notation and set builder notation.
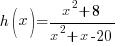
Solution:
Classify the Function
| Polynomial Function
A polynomial function is a function of the form
where n is a non-negative integer {0, 1, 2, 3, 4, …} and the coefficients |
|
| Rational Function
A rational function is a function of the form
where |
|
| Root Function (even index)
A root function is a function of the form
where n is an even positive integer greater than or equal to 2. |
The variable is inside or underneath a radical. The index of the radical is an even number. {2, 4, 6, 8, …} The square root is an even index although the index is not written. |
| Root Function (odd index)
A root function is a function of the form
where n is an odd positive integer greater than or equal to 2. |
The variable is inside or underneath a radical. The index of the radical is an odd number. {3, 5, 7, 9, …} The cube root is an odd index. |
Since the function 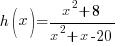 has a variable in the denominator and the numerator and denominator are polynomial functions this function is a rational function.
has a variable in the denominator and the numerator and denominator are polynomial functions this function is a rational function.
Find the Domain of a Rational Function
Division by zero is undefined. Having a zero as the denominator is equivalent to division by zero thus is also undefined. The rational function is undefined for any value of the variable that gives a zero denominator. Find these values by creating an equation to solve. The equation is the expression in the denominator equal to zero.
 |
|
| Solve the equation. This equation is a quadratic equation and can be solved by factoring, completing the square or the quadratic formula. |  |
| Solve by factoring. Factor the expression on one side. |  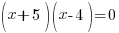 |
| Use the zero product property and set each factor equal to zero. | 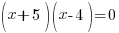 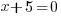 or or 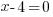 |
| Solve each equation. | 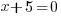 or or 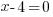 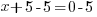 or or 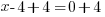 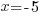 or or 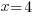 |
The values -5 and 4 give a zero value in the denominator, make the function undefined and must be excluded from the domain.
In set builder notation, the domain is 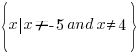
In interval notation, the domain is 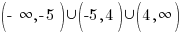
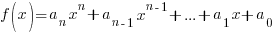
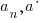 are from the real numbers.
are from the real numbers.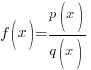
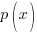 and
and  are polynomial functions and
are polynomial functions and 
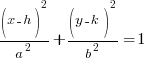
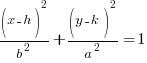
 . If
. If 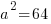 then
then 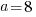 .
.
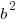 . If
. If 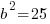 then
then  .
.

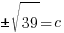
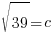 .
. above and below the center.
above and below the center.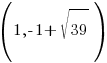 and
and