Category Archives: 3.4 Transformation of Functions

3.4 Transformation of Functions, 3.5 Graphing Techiques: Transformations

Graphing by Transformations: Quadratic

Example: For the function below.  Graph using transformations.

f(x)=-(x-3)^2+4

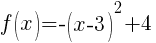
First we must examine the base function y=x^2

Graph using plotting points.  We can use the standard set of x-values to find ordered pairs.  Substitute the standard set of x-values into the base function to get the base graph.

xy
-2(-2)^2=4
-1(-1)^2=1
0(0)^2=0
1(1)^2=1
2(2)^2=4

The graph below shows the points plotted and the line that connects them.  The domain is 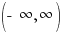(- infty, infty)  and the range is [0, infty)

 

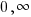Analyze the transformations.

f(x)=-(x-3)^2+4

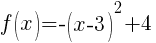
The -3 inside the square shifts the graph right 3 units.

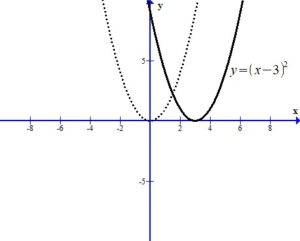
The – in the front of the base reflects the graph over the x-axis.

 

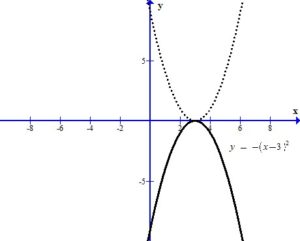
The +4 outside the square shifts the graph up 4 units.

You can see the graph after the transformations.

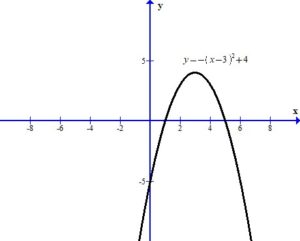
The domain is 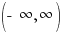(- infty, infty)  and the range is (- infty, 4]

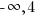Here is a video example of a transformation of a square function.