Category Archives: Finding the Equation of a Line
Finding the equation of a line perpendicular to another line
Example: Write the equation of a line in point-slope form passing through the point 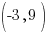 and perpendicular to the line whose equation is
and perpendicular to the line whose equation is 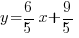 .
.
Solution:
Use the point-slope formula of the line to start building the line. m represents the slope of the line and 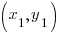 is a point on the line.
is a point on the line.
Point-slope formula: 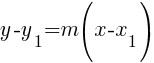
Although the slope of the line is not given, the slope can be deducted from the line being perpendicular to 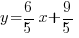 .
.
Perpendicular lines have negative reciprocal slopes. Since the slope of the given line is 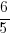 , the slope of the perpendicular line
, the slope of the perpendicular line 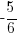 .
.
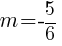 and
and 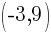
Substitute the values into the point-slope formula.

The point-slope form of the line is as follows.
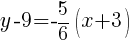
Example: Find the equation of a line perpendicular to another line and passing through a specific point. (The other line in slope intercept form)
Example: Find the equation of a line perpendicular to another line and passing through a specific point. (The other line in slope standard form)
Example: Find the equation of a line perpendicular to the x-axis.
Example: Find the equation of a line perpendicular to the x-axis and perpendicular to the y-axis.
Finding the Equation of a Line parallel to another line
Example: Find the equation of the line parallel to another line and passing through a specific point. (parallel equation in slope intercept form)
Example: Find the equation of the line parallel to another line and passing though a specific point. (parallel line in standard form)
Example: Find the equation of the line parallel to the x-axis or y-axis and passing through a specific point.
Example: What is an equation parallel to the y-axis?
Example: What is an equation parallel to the x-axis?
Finding the Equation of a line given a fractional slope and a point
Example: Find the equation of a line in slope intercept form given the slope of the line is 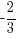 and the line passes through the point
and the line passes through the point 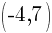
Solution:
Use the point-slope formula of the line to start building the line. m represents the slope of the line and 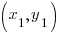 is a point on the line.
is a point on the line.
Point-slope formula: 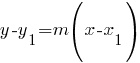
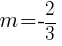 and
and 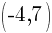
Substitute the values into the formula.
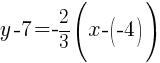
Since the instructions ask to write the equation in slope intercept form 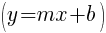 we will simplify and write the equation with y by itself on one side. I will also use the clearing fractions method to avoid having to add fractions.
we will simplify and write the equation with y by itself on one side. I will also use the clearing fractions method to avoid having to add fractions.
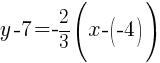
![3(y-7) = 3[-{2/3}(x+4)] 3(y-7) = 3[-{2/3}(x+4)]](https://math15fun.com/wp-content/plugins/wpmathpub/phpmathpublisher/img/math_980_0233a6f7265fc4d6a65d52d25e31bbd1.png) (Multiply by LCM)
(Multiply by LCM)
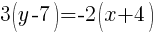 (Cancel Denominator)
(Cancel Denominator)

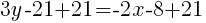
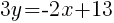
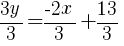
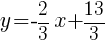
The equation of a line in slope intercept form with a slope of 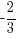 and passing through the point
and passing through the point 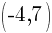 is
is 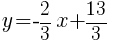
Finding the Equation of a Line given two points on the line
Example: Find the equation of a line in slope intercept form given the line passes through the two points 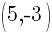 and
and 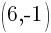 .
.
Solution:
First find the slope of the line.
Choose one of the points to be  and choose the other point to be
and choose the other point to be 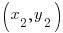 .
.
I will choose 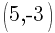 to be
to be  and choose
and choose  to be
to be 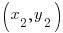 .
.
Substitute these values into the slope formula and simplify.
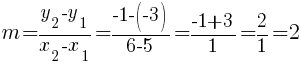
The slope of the line containing the points 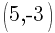 and
and  is
is 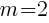 .
.
Then, use the point-slope formula of the line to start building the line. m represents the slope of the line and you can use 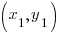 or
or 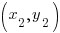 as the point on the line.
as the point on the line.
Point-slope formula: 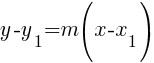
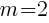 and
and 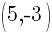
Substitute the values into the formula.
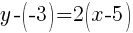
Since the instructions ask to write the equation in slope intercept form 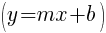 we will simplify and write the equation with y by itself on one side.
we will simplify and write the equation with y by itself on one side.
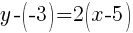


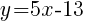
The equation of a line in slope intercept form passing through the two points 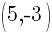 and
and 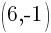 is
is 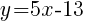 .
.
Finding the Equation of a Line given the slope and a point
Example: Find the equation of a line in slope intercept form given the slope of the line is 7 and the line passes through the point 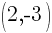
Solution:
Use the point-slope formula of the line to start building the line. m represents the slope of the line and 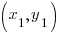 is a point on the line.
is a point on the line.
Point-slope formula: 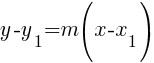
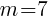 and
and 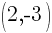
Substitute the values into the formula.
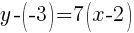
Since the instructions ask to write the equation in slope intercept form 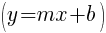 we will simplify and write the equation with y by itself on one side.
we will simplify and write the equation with y by itself on one side.
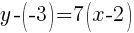
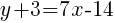
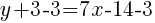

The equation of a line in slope intercept form with a slope of 7 and passing through the point 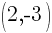 is
is  .
.
Example: Find the equation of the line.